Quantum Mechanics, The Uncertainty Principle, Schrodinger's Cat, & Other Misconceptions
By Jon Therkildsen, MSc MBA from University of Århus (2004)
THIS ARTICLE WILL ADDRESS AND ANSWER THE FOLLOWING:
WHAT IS AN ATOM?
WHAT IS A QUANTUM MECHANICAL WAVE FUNCTION?
WHAT IS SUPERPOSITION?
WHAT IS QFT?
WHAT IS THE OBSERVER EFFECT?
WHAT IS SCHRODINGER’S CAT?
WHAT IS THE UNCERTAINTY PRINCIPLE?
The study of Quantum Mechanics is quite complex, and yet it has found its way to the popular media and mainstream culture. Its marvelous wonders have encaptivated the minds of many. Unfortunately, there is much misinformation or fundamental inaccuracies out there about several of the more popular quantum mechanical theories. I will try to explain some of them here - brief, simple, and to the point, accurate enough to avoid the more general misinformation I often meet.
WHAT IS AN ATOM?
There is a beautiful story here. It is about how a simple thought can grow into perhaps the most important concept in Science today.
The idea that all matter is made up of tiny indivisible particles goes back two and half millennia to the Greek philosopher Leucippus of Miletus and his pupil Democritus of Abdera.
Now, of course, you may wonder; how on Earth could someone like Leucippus get such a profound idea when sliced bread was not even invented (took another 2 400 years, btw)? The answer is simple and, I think, beautiful. It was logic.
He wondered (and I am paraphrasing);
“… what if we were to break a stick. And then break one of its halves, and again break one of its halves and continue to do so for as long as it would be possible. Eventually, we should get such a tiny piece of the stick that we cannot - not even in principel - break it any further...”
He envisioned that all matter fundamentally (by the purest meaning of the word) consists of a collection of smaller indivisible particles or pieces. And incidentally, “atom” is Greek and means “indivisible” or “uncuttable” for this very reason.
Beautiful, isn’t it?
By simple deductive logic, he laid down the theory of atom and particle physics. And he did so to the extent that he (or rather Democritus) speculated that all of the Universe consisted of only atoms and empty space, and nothing else. Bold statement.
Today we call this “The Standard Model of Particle Physics.” We know of 17 such fundamental particles that, when grouped correctly, define all matter. They are the final piece of anything, including Leucippus’ stick. These 17 fundamental particles cannot be broken any further. They are indivisible. They are uncuttable. They are atoms.
Now indeed, the informed will note that “Atoms” are not understood as fundamental particles, and in fact, “Atoms” are a collection of the fundamental particles themselves (quarks, leptons, and bosons). While this is true, it is only true because we once thought what we called an “Atom,” was indeed what Leucippus also called an "Atom." Since then, we have learned that even Atoms can be subdivided into protons and neutrons, and those again can be subdivided further into our list of the 17 particles I reference above.
We call those fundamental particles or elementary particles, but a rose by any other name is still a rose. And the fundamental particles are atoms in every sense of the way Leucippus and Democritus meant them to be.
So what is Quantum Mechanics then?
Well, it is the science that describes and explains the nature and behavior of matter and energy on the atomic and subatomic levels. The mechanics of these smallest constituents of reality is quantum mechanics.
The Quantum Mechanical Wave Function, The SUPERPOSITION, & THE COLLAPSE
Quanta, or quantum systems, is a commonly used label for the tiniest constituents of reality, whether atoms or subatomic particles like those mentioned above. However, quanta is not a particularly informative term; it neither intuitively nor figuratively conveys what we are truly dealing with.
What’s peculiar about quanta is that they don’t behave quite like we would expect particles - or even waves - to behave. They seem to act like something else entirely.
A particle is often, though inaccurately, imagined as a tiny grain of sand. Add enough, and we can build a sandcastle. Quanta, however, are not like grains of sand. They are more akin to a sea of floating possibility, or a fuzzy cloud of possibility, if you can picture that.
The behavior of these quanta is best described by the so-called wave function (denoted Ψ), a mathematical concept originating from the work of Erwin Schrödinger and Max Born that describes the fundamental nature of reality. Unlike grainy particles fixed in space, quanta exist in a superposition, with probabilities of being found across a range of positions. Picture a cloud, thicker in some spots than others. These dense patches show where the particle is most likely to appear when measured, but before that, the entire cloud represents the quantum system. It’s not pinned to one spot; it spreads over a region, like an electron in an atom or a particle in an experiment, with varying probabilities.
In principle, a quantum system’s wave function can have a non-zero amplitude across all of space, meaning there is some probability (however small) of the superposition becoming a position anywhere in the universe, or even across the multiverse. However, in practice, superposition is typically local. The wave function tends to have significance within a finite region, constrained by physical dimensions such as potential wells, boundary conditions, or the experimental setup. A system may “involve the universe” through entanglement with distant systems or in interpretations like Many Worlds, where measurement branches the universe. Nevertheless, for practical purposes, picture the wave function as a localized cloud, ready to condense into one position when measured, like a drop of water forming from mist.
And so cloudy quanta may seem, yet they can build a sandcastle, even though they are neither grains of sand nor anything else easily imaginable in their natural state. This, naturally, contradicts our everyday experience here in the macroscopic world, where things are either one or the other, never both. You are either dead or alive, not both. You are in Paris or New York, not both. The wave function underlies this classical behavior, which emerges through decoherence and statistical mechanics. However cloudy it may seem, it describes our base reality impeccably.
You might wonder how we can think of quanta as “particles” within larger structures if they exhibit multiple states simultaneously and are inherently fuzzy in nature.
It is only through observation, measurement, or interaction that a quantum system assumes one definite quality over another. Werner Heisenberg described this transition as the shift from the possible to the actual. This phenomenon is commonly referred to as collapse. In that moment, the superposition yields a specific outcome, such as a particular position, momentum, or spin. The system, so to speak, “becomes” a grain of sand only when the wave function collapses. In more technical terms, this refers to any subatomic interaction in which a quantum system in superposition becomes entangled with its environment. It can be any interaction - dumb, smart, or dull, matters not. When such an interaction occurs, we observe what is called the collapse of the wave function - a non-unitary process in the Copenhagen interpretation.
Crucially, the wave function doesn’t become something else when it collapses; it remains a wave function but shifts into a specific state, called an eigenstate, tied to the property being measured, like position or energy. After this shift, if the system is left undisturbed, its natural energy dynamics cause the eigenstate to evolve, often forming a new superposition over time (governed by a concept called the Hamiltonian). This dance of collapse and evolution is what makes quantum mechanics so elusive. Through it all, the quantum system never stops being described by a wave function. In my view, this subtle but foundational aspect is one of the parts of quantum mechanics that many people find hard to grasp.
In the broader context, the true meaning of quantum collapse is still debated and explored through the various established interpretations of quantum mechanics. The most well-known is the Copenhagen interpretation, followed by the more speculative Many-Worlds interpretation. In fact, there are more than fifteen distinct interpretations, each offering a vastly different framework for the experimental work. Yet, whichever interpretation one subscribes to, it changes nothing in practice. They all come from the same underlying mechanics and the same body of evidence - they all work. In that sense, they are all equally correct. It does not show any unsureness or conflict with the macro worlds of sandcastles or cats. It shows that there is still much to be learned, but what we do know (or claim to know) about it cannot be described as an effect of an inherent unknowability. It is rather a quality of the quantum realm reality. It is, simply put, different down there.
To complicate matters further, everything in the quantum realm can be described entirely in terms of waves or entirely in terms of particles - whichever one prefers. In 1926, Erwin Schrödinger demonstrated the equivalence of wave and matrix mechanics, and Paul Dirac unified the formalism further in 1930. Niels Bohr pondered whether quanta might be neither waves nor particles, nor both. He further emphasized that the wave and particle aspects are mutually exclusive, yet both are necessary for a complete model. One alternative way to frame this connection or perhaps disconnection between these two terms is this:
Quanta travel like waves and arrive like particles.
I do not recall who is credited for describing it so (perhaps Bohr), but the term “like” is critical here, as the problem lies in the fact that our theories offer no account of what happens between the “transition from the possible to the actual.” Experiments reveal the presence of both waves and particles, but never the transition itself. No experiment has ever caught a wave function in the act of collapsing. Leslie Ballentine even argues that there is no collapse at all. Schrödinger himself once hoped to illuminate what occurs in that elusive in-between state, but never did, and for good reason: The point I’m getting at is this: the wave function describes the nature of quanta and quantum systems accurately. Schrödinger eventually came to see it not just as math: it was the real deal, describing quanta’s every move. They are not particles. They are not waves. They are wave functions - dancing to the tune of their quantum environment.
There is no better description, and it is an accurate description. However, and if we have to get anal about it, the wave function does not reside in ordinary Euclidean space; it lives in Hilbert space, a mathematical framework that represents the true domain of quanta. Any article attempting to convey quantum behavior owes at least a mention of this detail.
The takeaway, I hope, is this: the tiniest constituents of our reality are not particles, nor are they classical waves. They are fluctuating wave functions - a kind of indefinable third category that lies somewhere beyond both. Impossible for us to imagine, but possible for us to describe. The truth is, neither “wave” nor “particle” is an adequate label for what these entities truly are. Yet these are the metaphors we reach for when attempting to describe, in familiar terms, something that resists all intuition.
Beyond the elegant precision of mathematics, our language falters.
A more fitting label would simply be to call them what they are: wave functions. And then, perhaps, this is how we should refer to them:
Meticulous, the above description may be, but it is not very satisfying when writing an essay. Alas, we continue to call them particles or waves or both, depending on what we are trying to communicate. Neither, unfortunately, are correct, but often correct enough.
A wave function. Un-observed. Un-collapsed. A true representation of our TINIEST constituents
THE OVERARCHING THEORY OF QUANTUM MECHANICS
The best overarching theory of Quantum Mechanics we have today is the so-called “Quantum Field Theory” (QFT), which essentially collects several theories and theorems under a common conceptual framework, viewing all of reality as propagating fields.
To understand the framework of QFT, imagine reality as a vast ocean, not of water, but of invisible fields - one for each of the 17 fundamental particles in the Standard Model, like the electron field, the quark field, or the photon field. These fields are everywhere, permeating all of space, even in a perfect vacuum. They’re not made of stuff in the way we think of sand or water; they’re more like a set of possibilities, ready to ripple or vibrate.
Now, what we call a “particle” - say, an electron - isn’t a tiny dot floating in space. It’s a localized ripple or excitation in the electron field. Picture dropping a pebble into a pond: the ripple is the electron, but the pond itself is the field. When the field vibrates with a certain energy, that vibration behaves like a particle with properties such as mass or charge. This is why QFT is so powerful - it shifts our thinking from imagining quanta as grains of sand to seeing them as dynamic disturbances in a universal medium.
To come full circle, these fields are governed by wave functions, just like the quanta we discussed earlier. The wave function in QFT describes the probability of a field producing a particle at a given point in space and time. So, when we say a particle is “created” or “detected,” it’s the field’s wave function collapsing (or interacting) to produce a measurable outcome. This ties directly to our earlier point: quanta aren’t particles or waves in the classical sense; they’re manifestations of fields, dancing to the tune of their wave functions.
QFT takes Leucippus’ stik and elevates it to a new level. The “atoms” of today aren’t just particles; they’re the quantized vibrations of fields that span the universe. It’s a mind-bending shift, but it’s why your sandcastle, your cat, and even the stars are all ripples in the same cosmic ocean.
A DASH OF OBSERVER EFFECT
“The Observer Effect” is a term frequently used in quantum mechanics, often portrayed with more drama than accuracy. Let’s clarify things a bit. This term describes how measuring one property of a quantum system, such as a particle’s position, inevitably disrupts another, like its momentum. This phenomenon doesn't involve a mystical observer or a conscious mind peering into reality. It's simply physics in action. The effect originates from Heisenberg’s Uncertainty Principle.
Consider an electron: to measure its position, you might bounce a photon off it, akin to flicking on a cosmic flashlight. However, this photon inevitably gives the electron a tiny push, disturbing its momentum. If you use a gentler photon to reduce this disturbance, you get a less precise measurement of its position. This disturbance isn't due to flaws in our instruments; rather, it’s a fundamental characteristic of quantum mechanics, where every measurement is inherently an interaction. No human eyes or conscious thoughts are required - a detector’s click alone suffices.
Now, here's where things become nuanced: the Observer Effect is frequently mistaken for wave function collapse, though they are distinct concepts. Wave function collapse describes when a quantum system, like Schrödinger’s Cat, transitions from a fuzzy superposition of possibilities into a single definite state, such as a specific location. Conversely, the Observer Effect concerns the inevitable interference that measurement causes to related properties, regardless of whether collapse occurs. For instance, measuring an electron’s position with a photon disturbs its momentum, but this doesn’t necessarily collapse its wave function.
In the renowned double-slit experiment, determining through which slit an electron travels alters its wave-like behavior, creating a particle-like pattern. The crucial factor here is entanglement, not observation per se: measurement entangles the electron with the detector, leading to collapse, with observation simply facilitating the interaction. Popular media often muddle these distinctions, depicting conscious minds shaping reality, but this misrepresents the theories.
While philosophers continue debating the deeper implications of the "observer" in quantum mechanics, the Observer Effect itself remains a firmly established and fully understood component of quantum theory and experimentation.
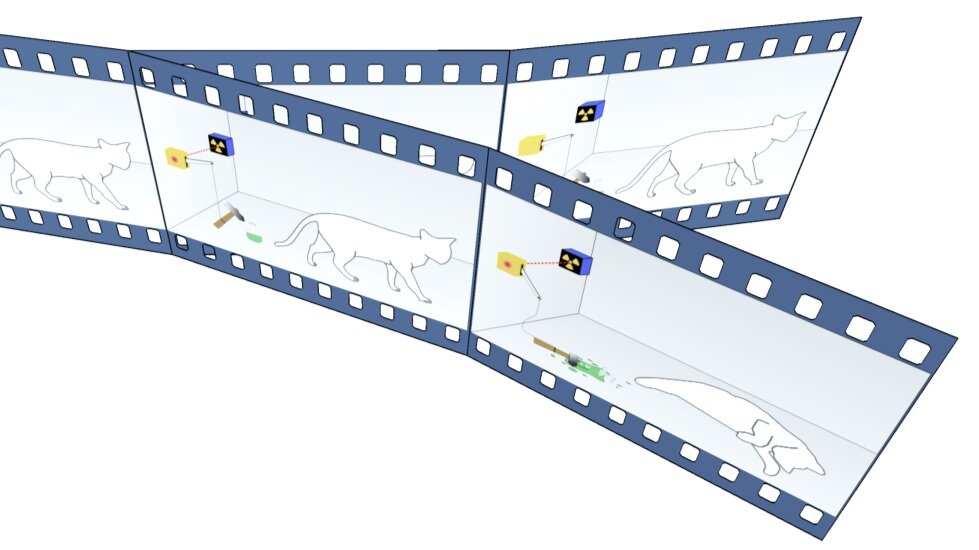
Schrödinger's Cat
Schrödinger’s Cat, the iconic thought experiment, illustrates the strangeness of quantum superposition under the Copenhagen interpretation. It imagines a cat sealed in a box, its fate tied to a single radioactive atom. The atom exists in a superposition of decayed and non-decayed states, and - through a mechanism involving poison - the cat becomes entangled with that superposition, enabling the poison to be released and not-released. Until the system is measured, quantum mechanics suggests the cat is therefore both alive and dead.
This isn’t about literally proving a cat can exist in two states, but about exposing the tension between quantum rules and our classical intuitions. Subatomic particles like radioactive atoms do, in fact, exist in superpositions of all possible states until, for example, measurement triggers a collapse. In this setup, the atom’s indeterminacy is transferred to the macroscopic level of the poison being released or not, resulting in a paradox: the cat is not either alive or dead; it is both, simultaneously.
More precisely, the cat’s fate is not 50/50. It is 100% alive and 100% dead until observed, when it only then becomes one or the other. This conclusion defies classical logic, which permits only one reality at a time.
Interestingly, Erwin Schrödinger stands at the heart of both the wave function (for which he was awarded the Nobel Prize) and the thought experiment bearing his name, which was designed to ridicule the bizarre consequences of that very wave function he helped author.
Schrödinger devised this thought experiment not to endorse but to critique his wave function. He wanted to demonstrate that if taken literally at all scales, quantum mechanics leads to absurd conclusions and so indicates something is amiss. Nearly a century later, however, a wealth of experimental evidence has supported the accuracy of his wave function, thereby undermining this original objection.
Since then, we’ve come to understand that the tiniest constituents of reality, as mathematically described by the wave function, are neither classical waves nor particles. Rather, they represent a distinct kind of mathematical reality - one that defies analogy to our everyday macroscopic experience. And yes, that includes cats. Moreover, the term “observation” in quantum mechanics cannot be simplistically equated with “opening the box to take a look.” In reality, any subatomic interaction, regardless of a conscious observer, can constitute a collapse. This subtlety further undermines the literal macro framing of Schrödinger’s experiment.
In the end, Schrödinger’s Cat serves as a powerful illustration of just how deeply counterintuitive quantum mechanics can be. While Schrödinger - in passionate unison with Einstein, by the way - sought to challenge or even discredit aspects of quantum theory through such paradoxes, the thought experiment ultimately underscores the coherence of quantum mechanics rather than refuting it.
IN THE MANY-WORLDS INTERPRETATION, THE CAT LIVES IN ONE WORLD AND DIES IN ANOTHER
A competing view to the more classical Copenhagen interpretation, in which the cat is either dead or alive, is the so-called Many-Worlds interpretation, which offers a radically different solution to Schrödinger’s thought experiment. In this view, the cat is 100% dead and 100% alive, but in parallel worlds. According to this interpretation, the wave function never truly collapses. Instead, it splits into multiple world-branches, each representing a different outcome.
So, in one world, you are dead; in another, alive. You are in Paris in one branch, and in New York in another. The cat dies in one universe and continues to live in another. We won’t know which of these realities we inhabit until we open the box, aka until we observe. In that sense, the Many-Worlds interpretation satisfies the paradox without contradicting our classical experience of reality. It is, arguably, a more earnest and uncompromising reading of the wave function - one that refuses to arbitrarily select a single outcome.
We cannot say with certainty which interpretation is “correct,” and there are many others beyond these two. One could argue it doesn’t much matter, since the wave function holds regardless. The empirical predictions are the same, and the mathematics remains untouched. In that sense, both interpretations are correct, though, ironically, they can’t both be.
In any case, the Many-Worlds view posits that the cat is alive in one universe and dead in another. And the truth is, we won’t know which world we’re in until we observe it.
The Uncertainty Principle
Heisenberg’s Uncertainty Principle is often misrepresented as implying that nature is inherently unpredictable.
This would be false.
The principle has nothing to do with mysticism or randomness; rather, it reflects a fundamental feature of how nature operates at the quantum level. At its core, the Uncertainty Principle states that certain pairs of properties - such as position and momentum - cannot simultaneously be known with arbitrary precision. This limitation does not stem from flaws in our measurement tools. It arises directly from the wave-like nature of quantum systems. Quanta are not grains of sand, with a definite momentum and position; they are something else - a mundane explanation to this principle it may be, but this is the gist.
Interestingly, Werner Heisenberg himself described the concept using terms like Ungenauigkeit (inexactness) and Unbestimmtheit (indeterminacy). His mentor, Niels Bohr, preferred Unsicherheit (unsureness). Today, the German term Unschärfe - often translated as "blurredness" or "fuzziness" - is widely used, perhaps most fittingly so. It captures not only the essence of the principle, but something more profound: the intrinsic character of nature itself.
A common confusion, I suspect, stems from trying to reconcile how the probabilistic fuzziness of the wave function gives rise to the certainties of everyday objects. Take a chair or a sandcastle: both are composed of countless particles, each governed by their own uncertainties. And yet, they form stable, tangible, and predictable structures. Why? Because, while individual particles behave in unpredictable fuzziness, their aggregate behavior averages out, yielding the deterministic world we experience. I know exactly where my chair is and how fast it might be moving, and yet it is slave to the principle like all other matter is.
An analogy I like to use is that of flipping a coin. The chance of landing heads is 50%. But flip twice, and you might get tails both times. Flip ten times, and maybe heads comes up only twice. Flip a hundred times, and you might get 48 heads. It’s close, but still off by the expected distribution. However, flip a gazillion times, and the distribution approaches exactly 50/50. This phenomenon is known as The Law of Large Numbers, which is how probability functions can produce certain results. This is how fuzzy becomes solid.
Though each is fuzzy and uncertain on its own, together they form the solid, predictable fabric of the world we know.
Our reality is built up of a gazillion probability functions.
To sum up
Nature (all matter and energy) consists of indivisible particles. We know of 17 elementary particles. They are, however, not particles nor waves. They are wave functions that exist in a superposition of all possible states simultaneously. When observed or interacted with, their wave function collapses to a definite state, like a specific position, with probabilities set precisely by the wave function. Not, ambiguously or approximately, but accurately and absolutely.
Far from speculation, these truths have been confirmed by countless experiments, standing beyond any reasonable doubt. From misty clouds of possibility to the sandcastles of reality, quanta is what we all are.
That is pretty much it. Easy.
Photos via Google